Στοιβάζοντας κανονικά Οκτάγωνα
- Details
- Category: Εφαρμογές μαθηματικών
- Published on Wednesday, 15 October 2014 23:23
- Written by Super User
- Hits: 1014
Η τοποθέτηση αντικειμένω σε ένα χώρο, ώστε να γεμίσει αυτός όσο καλύτερα και περισσότερο γίνεται είναι ένα από τα ιδιαίτερα σημαντικά προβλήματα υπάρχει μεγάλο εύρος στη βιβλιογραφία για αυτό.
Ωστόσο, όταν η τοποθέτηση αφορά τη στοίβαξη περισσότερο κανονικών αντικειμένων, τότε αυτό μπορεί να μελετηθεί ως αυστηρά μαθηματικό πρόβλημα.
Στη συνέχεια ακολουθεί μία μετάφραση (με λίγες επεξηγηματικές πρόσθηκες του άρθρου Packing Regular Octagons από το blog της American Mathematical Society.
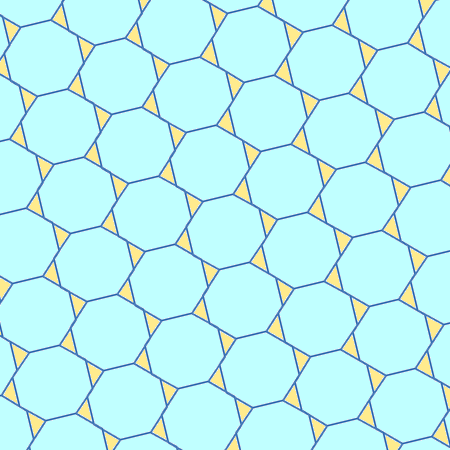
Στο προηγούμενο σχέδιο φαίνεται η πυκνότερη στοίβαξη (εννοούμε τη στοίβαξη η οποία αφήνει τα μικρότερα κενά στο επίπεδο) κανονικών οκταέδρων στο επίπεδο, όπως σχεδιάστηκε από τον Graeme McRae. Είναι ενδιαφέρουσα διότει αποτελεί ένα αντιπαράδειγμα του 2-διάστατου αναλόγου μίας εικασίας που έκανε ο Stanislaw Ulam (Πολωνο-Αμερικανός Μαθηματικός 1909-1984), της Εικασίας Στοιβάγματος του Ulam,
σύμφωνα με την οποία από όλα τα κυρτά σώματα (περιγραφικά σώματα που δεν έχουν πτυχές ή μαθηματικά σώματα στα οποία κάθε ευθύγραμμο τμήμα με άκρα μέσα στο σώμα αυτό βρίσκεται ολόκληρο επίσης μέσα σε αυτό το σώμα) στον 3-διάστατο Ευκλείδιο χώρο η σφαίρα έχει την μικρότερη μέγιστη δυνατή πυκνότητα ταξινόμησης. Μάλιστα για όμοιες σφαίρες αυτή η πυκνότητα είναι
This is the densest packing of regular octagons in the plane, drawn by Graeme McRae. It is interesting because it is a counterexample to the 2-dimensional analogue of a conjecture made in 3 dimensions by Stanislaw Ulam.
Ulam?s packing conjecture states that of all convex bodies in 3d Euclidean space, the ball has the smallest maximum packing density. Since congruent balls can be packed with a density of
- See more at: http://blogs.ams.org/visualinsight/2014/10/15/packing-regular-octagons/?utm_content=buffer2d542&utm_medium=social&utm_source=facebook.com&utm_campaign=buffer#sthash.InAkQTlt.dpufΕπιπλέον, η πρώην εικασία του Kepler , η οποία έχει πλέον αποδειχθεί και ισχύει ως θεώρημα, αναφέρει ότι καμία διάταξη ισομεγεθών σφαιρών δεν έχει μεγαλύτερη μέση πυκνότητα από αυτήν:

Η πυκνότητα της οποίας είναι ελαφρά μεγαλύτερη του 74%.
Η εικασία του Ulam λέει ότι για οποιοδήποτε άλλο κυρτό σώμα στον Ευκλείδιο 3-διάστατο χώρο, μπορούμε να στοιβάξουμε όμοια αντίτυπά του με πυκνότητα μεγαλύτερη του .
Το αντίστοιχο πρόβλημα στις 2 διαστάσεις (στο επίπεδο δηλαδή) θα έλεγε ότι κάθε κυρτό σχήμα στο Ευκλείδειο επίπεδο μπορεί να στοιβαχθεί με πυκνότητα τουλάχιστον:
Παρόλα αυτά η πυκνότερη τοποθέτηση κανονικών εξαγώνων, που φαίνεται στο αρχικό σχέδιο, έχει πυκνότητα μόλις:
η οποία αν και είναι μόλις 0.7 χιλιοστά μικρότερα, αποτελεί επαρκές αντιπαράδειγμα για την αντίστοιχη εικασία στο 2-διάστατο χώρο.
Η στοίβαξη των οκταγώνων του Graeme McRae ισχυρίζεται ότι είναι η πυκνότερη δυνατή για κανονικά οκτάγωνα στο άρθρο εδώ.
Γενικότερα, οι επικαλύψεις χώρων με κανονικά σχήματα λέγονται και πλακοστρώσεις (Tilings) και μπορεί να βρει κανείς ενδιαφέρουσες περισσότερες πληροφορίες εδώ , εδώ και κυρίως εδώ .
This is the densest packing of regular octagons in the plane, drawn by Graeme McRae. It is interesting because it is a counterexample to the 2-dimensional analogue of a conjecture made in 3 dimensions by Stanislaw Ulam.
Ulam?s packing conjecture states that of all convex bodies in 3d Euclidean space, the ball has the smallest maximum packing density. Since congruent balls can be packed with a density of
and Kepler?s conjecture, now a theorem, says this is a best possible, Ulam?s conjecture says that for any other convex body in R3, we can pack congruent copies of this body with a density more than π/18???.
The analogous conjecture in 2 dimensions would say that every convex region in the Euclidean plane can be packed with a density at least
However, the densest packing of regular octagons, shown above, has density only
It density is only about .0007 less, but this suffices to refute the conjecture!
There is another more famous packing of regular octagons, the truncated octagonal tiling:
- See more at: http://blogs.ams.org/visualinsight/2014/10/15/packing-regular-octagons/?utm_content=buffer2d542&utm_medium=social&utm_source=facebook.com&utm_campaign=buffer#sthash.InAkQTlt.dpufThis is the densest packing of regular octagons in the plane, drawn by Graeme McRae. It is interesting because it is a counterexample to the 2-dimensional analogue of a conjecture made in 3 dimensions by Stanislaw Ulam.
Ulam?s packing conjecture states that of all convex bodies in 3d Euclidean space, the ball has the smallest maximum packing density. Since congruent balls can be packed with a density of
and Kepler?s conjecture, now a theorem, says this is a best possible, Ulam?s conjecture says that for any other convex body in R3, we can pack congruent copies of this body with a density more than π/18???.
The analogous conjecture in 2 dimensions would say that every convex region in the Euclidean plane can be packed with a density at least
However, the densest packing of regular octagons, shown above, has density only
It density is only about .0007 less, but this suffices to refute the conjecture!
There is another more famous packing of regular octagons, the truncated octagonal tiling:
- See more at: http://blogs.ams.org/visualinsight/2014/10/15/packing-regular-octagons/?utm_content=buffer2d542&utm_medium=social&utm_source=facebook.com&utm_campaign=buffer#sthash.InAkQTlt.dpufThis is the densest packing of regular octagons in the plane, drawn by Graeme McRae. It is interesting because it is a counterexample to the 2-dimensional analogue of a conjecture made in 3 dimensions by Stanislaw Ulam.
Ulam?s packing conjecture states that of all convex bodies in 3d Euclidean space, the ball has the smallest maximum packing density. Since congruent balls can be packed with a density of
and Kepler?s conjecture, now a theorem, says this is a best possible, Ulam?s conjecture says that for any other convex body in R3, we can pack congruent copies of this body with a density more than π/18???.
The analogous conjecture in 2 dimensions would say that every convex region in the Euclidean plane can be packed with a density at least
However, the densest packing of regular octagons, shown above, has density only
It density is only about .0007 less, but this suffices to refute the conjecture!
There is another more famous packing of regular octagons, the truncated octagonal tiling:
- See more at: http://blogs.ams.org/visualinsight/2014/10/15/packing-regular-octagons/?utm_content=buffer2d542&utm_medium=social&utm_source=facebook.com&utm_campaign=buffer#sthash.InAkQTlt.dpufThis is the densest packing of regular octagons in the plane, drawn by Graeme McRae. It is interesting because it is a counterexample to the 2-dimensional analogue of a conjecture made in 3 dimensions by Stanislaw Ulam.
Ulam?s packing conjecture states that of all convex bodies in 3d Euclidean space, the ball has the smallest maximum packing density. Since congruent balls can be packed with a density of
and Kepler?s conjecture, now a theorem, says this is a best possible, Ulam?s conjecture says that for any other convex body in R3, we can pack congruent copies of this body with a density more than π/18???.
The analogous conjecture in 2 dimensions would say that every convex region in the Euclidean plane can be packed with a density at least
However, the densest packing of regular octagons, shown above, has density only
It density is only about .0007 less, but this suffices to refute the conjecture!
There is another more famous packing of regular octagons, the truncated octagonal tiling:
- See more at: http://blogs.ams.org/visualinsight/2014/10/15/packing-regular-octagons/?utm_content=buffer2d542&utm_medium=social&utm_source=facebook.com&utm_campaign=buffer#sthash.InAkQTlt.dpuf